Energy transfer in rotationally inelastic collisions: a new scaling law based on time-energy uncertainty

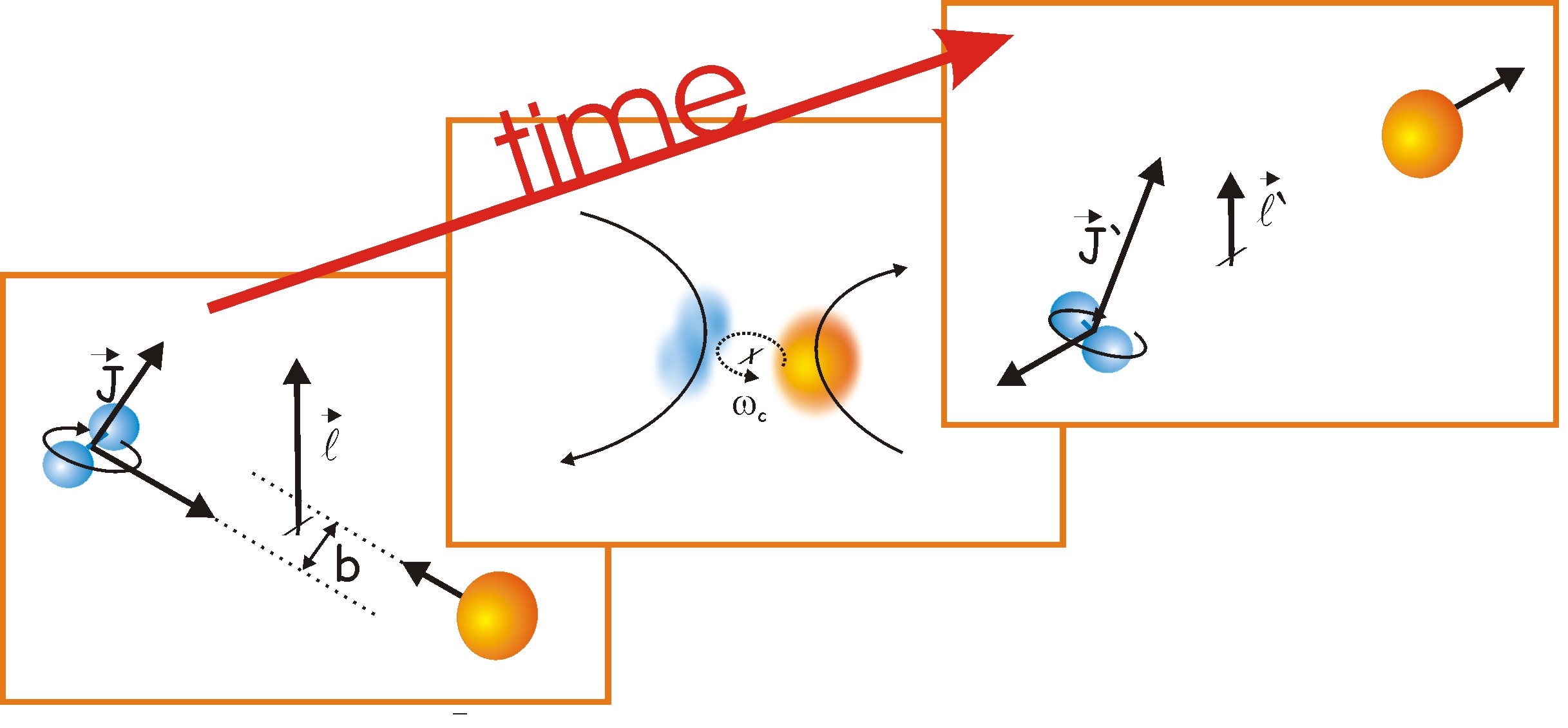
The dynamics underlying the collision process can experimentally only be determined to an accuracy given by Heisenberg´s time-energy uncertainty. Based on this assumption an effective angular momentum parameter lc = 2 ћ is derived that limits the possible amount of angular momentum transferred during a restricted interaction time [1,2]. This angular momentum transfer limit together with a competing energy gap term [3] is incorporated into the ECS theory [4] yielding a new scaling model for rotationally inelastic collisions: The Angular Momentum and Energy Corrected Sudden Approximation (AECS).

The AECS model is experimentally verified using time-resolved coherent anti-Stokes Raman spectroscopy (fs-CARS). We have investigated rotational energy transfer (RET) in N₂ induced by collisions with He, Ne, N₂, Ar and Kr, and additionally CO-CO as well as C₂H₂-C₂H₂ collisions. In all cases the experimental results are in excellent agreement with the AECS model (see Figures below). An central feature of the AECS scaling is the limitation of the number of free parameters to two in contrast to typically four or five in common ECS models using energy gap laws.

Figure: Left: Simulated transients using the ECS-P, ECS-E [6] and AECS models, respectively. At 5 bar significant deviations of the three models are found. Right:Experimental fs-CARS transients (symbols) obtained at room temperature for N₂ (500 mbar) colliding with rare gases (~ 4 bar). The transients obtained with the AECS model (solid lines) closely fit the experimental data

Figure: Linewidth data (symbols) for N₂-N₂ collisions between 295 and 1500 K are reported in Ref. [5]. The solid lines calculated with AECS and the model parameters obtained at room temperature with fs-CARS are in good agreement.

[1] P. Beaud and G. Knopp, Chem. Phys. Lett. 371 (2003) 194.
[2] G. Knopp, P. Radi, M. Tulej, T. Gerber and P. Beaud, J. Chem. Phys. ,118 (2003) 8223.
[3] P. Beaud, T. Gerber, P. Radi, M. Tulej, and G. Knopp, Chem. Phys. Lett., 373 (2003) 251.
[4] A.E. DePristo, S.D. Augustin, R. Ramaswamy, and H. Rabitz, J. Chem. Phys. 71 (1979) 850.
[5] L.A. Rahn and R.E. Palmer, J. Opt. Soc. Am. B 3 (1986) 1164.
[6] L. Bonamy and J.V. Buldyreva, Phys. Rev. A 63 (2000) 012715.

.